ResearchEd Rugby 2019
 Another Saturday in June and another education conference, and another gorgeous setting, this time in Rugby. The stairs are important – they’re the two spiral flights we went up and down to get to the maths room. Most times when I go to a conference, my exercise is considerably lacking on that day, but ResearchEd Rugby even managed to account for step rate too! There was a good maths line up, which is where I spent most of the day, but I did venture out to a couple of other sessions, so I’ll start with the one I was most excited to go to, and come onto the maths afterwards. (As always, apologies if I’ve misinterpreted anything, or just got it wrong).
Another Saturday in June and another education conference, and another gorgeous setting, this time in Rugby. The stairs are important – they’re the two spiral flights we went up and down to get to the maths room. Most times when I go to a conference, my exercise is considerably lacking on that day, but ResearchEd Rugby even managed to account for step rate too! There was a good maths line up, which is where I spent most of the day, but I did venture out to a couple of other sessions, so I’ll start with the one I was most excited to go to, and come onto the maths afterwards. (As always, apologies if I’ve misinterpreted anything, or just got it wrong).
Oliver Caviglioli
I first heard of dual coding from Peps McCrea, @PepsMccrea, a couple of years ago at the Maths and Science ResearchEd in Oxford. My takeaway then was within powerpoint presentations, and that the brain couldn’t process written text and speech at the same time, so it is better to have a visual on the presentation to go with the speech. This made complete sense, and since then, I’m much more aware in CPD sessions when there’s a large amount of text on the presentation, that I cannot read it whilst trying to listen properly to what is being said.
Last year I prepared some revision powerpoints for our year 10 form tutors, and dual coding came into play in two forms. Firstly, as a revision technique from the LearningScientists.org, as one of the six strategies for effective learning, but secondly in the design of the powerpoint. Similar, when I prepared CPD at the start of the school year about the challenges faced with reading and the new GCSEs, I was very conscience of dual coding, and tried to make the presentation as visual based as possible.
But now was the chance to hear from the expert, and as I tweeted, @olicav did not disappoint. Oliver’s session was very practical based, in that he presented two problems and a solution, with audience participation! I therefore didn’t write much in notes, but then Oliver has a very comprehensive website, olicav.com, where all his diagrams he presented are available (the quote below was also downloaded from there). Spoiler alert though, the summing up for me was:
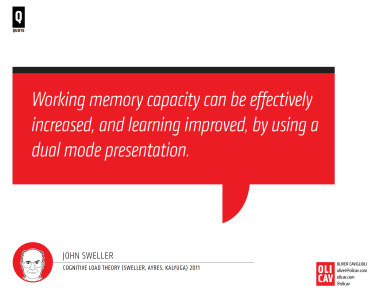
- Speech and text is linear
- Schema isn’t linear – we think in diagrams
- Text by itself has a computational inefficiency
- Visual and text are separate but associated, and using both together gives double the chance to process and remember (John Sweller’s dual mode presentation)
The example that Oliver used for the computational inefficiency of text greatly resonates with me for some of those longer maths questions where there is so much information to pick apart. I’m already very much a fan of a diagram or picture, but maybe I need to consider raising the importance of this earlier on for students, even spend time just trying to turn questions into diagrams as an exercise in itself.
The final part was our participation in a recount and redraw exercise. Oliver (as the teacher) modelled, via a mind map diagram, and explained, via speech, a history of Corsica. We, as the student, copied the diagram as Oliver was explaining it. The student then recounts the diagram to a peer, tracing the elements and elaborating on what they have written as they go. Finally, the student redraws the diagram from memory, noting that the retrieval practice would be even more effective if the student did this again a day later and a month later.
Mark Lehain
First of all, Mark is an absolute gentleman, and was very kind in giving his time after the presentation! Mark, @lehain, was talking about behaviour, and from the point of view of a Head Teacher, how teachers can help SLT. Some highlights that I took from Mark were:
- Parents and teachers for excellence – a group that believes that every school child should have an excellent education, and that the key characteristics of those schools that deliver this are excellent behaviour, a challenging curriculum, rigorous testing and enriching extra-curricular activities.
- Recommending Tom Bennet’s Creating a Culture, and in particular the 8 principles of designing a culture.
- Differences of opinion between SLT and teachers may be there because of different experiences, different values or different analyses of situations.
- If you asked “What is the point of education”, there would be different responses. So if we can’t agree on the why, then we’re probably not going to agree on the how. However, we need to disagree well (we’re all a team, working together for the students, and we all have feelings).
The point about disagreeing well particularly resonated for me in the way some educationalists respond on Twitter. I think I would respond so much more if we could all disagree well (or maybe I just need to be 10% braver)!
Don Steward
Now the maths bit! I love Don Steward’s resources. They are so thoughtful of the maths, the connections and the applications, so it’s a real pleasure to explore some maths with Don. Don took one of the Edexcel ratio questions from this year as a starting point to explore ratio. The question involved a ratio of counters, which when other counters (of both types) were added, the ratio changed. This was a lovely practical session, where it’s just great to have some time to explore some maths. We looked at the different methods to solve this question, and the links between. A snapshot of the session includes:
- Establishing what a ratio is (and it being ok to ask students the obvious questions)
- Using scaling to answer the question (testing it out with values and seeing what works)
- Introducing a multiplier to a ratio when introducing ratio (so 4:3 would be 4k:3k) and therefore allowing an algebraic solution
- Graphing ratios and linking to vectors to move from one graph to another – this developed could be developed into a vector method to solve the problem, and also links to a simultaneous equation method
- Developing the question to find the possible number of counters added to change the ratio, which led to sequences and nth term
- The work of Van Hiele, the avoidance of fractions and the proportion matrix
- The ICAAMS project, where year 8 students were given 2 questions, which both use the same method, but had a large variance in success rate.

I’ve been teaching proportion with year 8s this week, and a started using Don’s resource on proportion boxes, as I wanted students to be able to understand that proportion was a multiplicative relationship, and that relationship can go either way. So I’m going to give these two questions to my year 8s and hope that the work we’ve done will make some difference to their success rate with the first question compared to the second.
Jo Morgan
It is always a pleasure to listen to Jo, @mathsjem, on whatever topic she is talking about, because you can guarantee that she knows that topic in depth before she presents it. Jo also gives some great nuggets of practice or exploration and takeaways, which again her multiplication session delivered brilliantly. Highlights:
- A method for any single digit multiplication greater than 5×5 (and a chance to prove why it works).
- Jo’s thoughts on why timetables fluency is important (and I completely agree that although we can and do teach students how to work out timetables without having to know them off by heart, this is no good when the reverse is required, eg for simplifying fractions or factorising).
- A review of different multiplication methods from Smile’s “Multiplication makes sense” and an explanation as to why the Russian Peasant Method works.
- A presentation on the column method, and an argument against each of the reasons given for it to be a “formal written method”. This was not having a go at the column method, just the reasoning given at the time.
Thanks Jo!
Tom Francombe
I hadn’t heard Tom speak before, but I am more than familiar with his book, Practising Mathematics, that I have open on my desk at home pretty much constantly! As Naveen, being a victim of the train problems, was unable to make her session later on, Tom stepped in and did two sessions. Part one was more about the theory, whereas part two was a chance to try some practice.

Who knew there were so many types of practice? Although Tom started with deliberate practice, he explained how really this is unfeasible in the classroom, as it involves immediate feedback on mistakes and altering the practice in light of this. So his talk was more about purposeful practice, an in-between of naive practice and deliberate practice.
Tom talked about fade feedback, which I hadn’t heard of as a term before, but in practice are techniques that I do use in the classroom. As the term suggests, fade feedback reduces the level of feedback that a student receives when solving problems, but based on how the problem is prevented. So the structure could look like the following:
- Initially give the answers, in “show that…” style questions
- Then have answers available to match
- Structure questions so the answers have some sort of commonality (Don’s resources have some good examples of this)
- No answers available.
Tom also spoke about organising practice, comparing blocked practice and interleaving, and within the interleaving, either mixing different questions (such as multiplying fractions and finding areas of kites), or mixing topics within the question (finding areas of kites with lengths as fractions). This is an important area of contemplation for me at the moment. I use spaced practice in my BBQ starters for KS4, and my homework and quizzes for KS3, but I don’t interleave in “main” classwork, unless it’s part of revision. Yet, as Tom pointed out, in maths we have to choose our strategies before we use them, which is not addressed in blocked practice. This isn’t to say blocked practice doesn’t have a place, and Tom emphasised that with first learning, blocked practice would be more suitable. This is definitely an area I need to think more on!
 When presenting some of the possible ideas of practice tasks, Tom’s purpose was to find tasks that can develop fluency, but also allow for mathematical reasoning. He indicated that the research said that these tasks were as effective as straight forward exercise tasks, but they can be more motivating and enriching.
When presenting some of the possible ideas of practice tasks, Tom’s purpose was to find tasks that can develop fluency, but also allow for mathematical reasoning. He indicated that the research said that these tasks were as effective as straight forward exercise tasks, but they can be more motivating and enriching.  We had a go at a few of these; this Pythagoras task adds another dimension to your normal “square, square, add or subtract, square root” type questions, and the multiplication task (which I’ve used before) gave us chance to explore some reasoning.
We had a go at a few of these; this Pythagoras task adds another dimension to your normal “square, square, add or subtract, square root” type questions, and the multiplication task (which I’ve used before) gave us chance to explore some reasoning.
Pete Mattock
The great thing about attending one of Pete’s session (as well as his presentation) is that we get to play! This time, Pete, @MrMattock, brought his algebra tiles along. I’m very much a newbie with manipulative, only really having access to multilink cubes. I did make my own +1/-1 counters this year for teaching negative numbers, which I loved using!
However, Pete’s session wasn’t actually about manipulative, they were just a bonus. Pete was talking about the concepts of Teaching for Mastery (as described by the NCETM), Rosenshine’s Principles of Instruction and Sweller’s work on Cognitive Load Theory. Pete suggested that rather than look at them as three separate entities, we can find the overlaps between them, as then we’d be looking at the overlaps between high performing jurisdictions (Teaching for Mastery), high performing teachers (Rosenshine’s Principles of Instruction) and how the memory works (Cognitive Science).
I made the mistake here of listening and doing so much, that I didn’t write any notes! Pete gave some lovely examples of how these three concepts (is that the right word to describe them?) are used together, but I don’t trust myself to recall these accurately enough to describe (sorry Pete!).